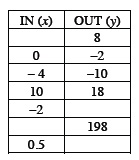
x-intercept(s)
x-coordinates of x-intercepts. For example, we might say that the x-intercepts of the graph below are (0, 0) and (2, 0), or we might just say that the x-intercepts are 0 and 2.
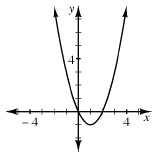
x → y table
An x → y table, like the one at right, represents pairs of values of two related quantities. The input value (x) appears first, and the output value (y) appears second. For example, the x → y table at right tells us that the input value 10 is paired with the output value 18 for some rule.