algebra tiles
area
For this course, area is the number of square units needed to fill up a region on a flat surface. In later courses, the idea will be extended to cones, spheres, and more complex surfaces.
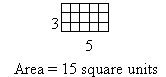
Associative Property of Addition
| A | B | C | D | E | F | G | H | IJK | L | M | N | O | P | Q | R | S | T | UV | WX | YZ |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Algebra Connections Glossary | ||||||||||||||||||||
absolute value |
|
|---|---|
| The absolute value of a number is the distance of the number from zero. Since the absolute value represents a distance, without regard to direction, it is always non-negative. Thus the absolute value of a negative number is its opposite, while the absolute value of a non-negative number is just the number itself. The absolute value of x is usually written “| x |”. For example, | −5 | = 5 and | 22 | = 22. |
|
Additive Identity Property |
|
| The Additive Identity Property states that adding zero to any expression leaves the expression unchanged. That is, a + 0 = a. For example, −2xy² + 0 = −2xy². | |
Additive Inverse Property |
|
| The Additive Inverse Property states that for every number a there is a number −a such that a + (−a) = 0. For example, the number 5 has an additive inverse of −5; 5 + (−5) = 0. The additive inverse of a number is often called its opposite. For example, 5 and −5 are opposites. | |
Additive Property of Equality |
|
| The Additive Property of Equality states that equality is maintained if the same amount is added to both sides of an equation. That is, if a = b, then a + c = b + c. For example, if y = 3x, then y + 1.5 = 3x + 1.5. | |
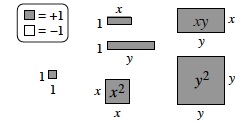
algebra tiles |
|
| An algebra tile is a manipulative whose area represents a constant or variable quantity. The algebra tiles used in this course consist of large squares with dimensions x-by-x and y-by-y; rectangles with dimensions x-by-1, y-by-1, and x-by-y; and small squares with dimensions 1-by-1. These tiles are named by their areas: x², y², x, y, xy, and 1, respectively. The smallest squares are called “unit tiles.” In this text, shaded tiles will represent positive quantities while unshaded tiles will represent negative quantities.
|
|
area |
|
For this course, area is the number of square units needed to fill up a region on a flat surface. In later courses, the idea will be extended to cones, spheres, and more complex surfaces.
|
|
Associative Property of Addition |
|
| The Associative Property of Addition states that if a sum contains terms that are grouped, the sum can be grouped differently with no effect on the total. That is, a + (b + c) = (a + b) + c. For example, 3 + (4 + 5) = (3 + 4) + 5. | |
Associative Property of Multiplication |
|
| The Associative Property of Multiplication states that if a product contains terms that are grouped, the product can be grouped differently with no effect on the result. That is, a(bc) = (ab)c. For example, 2 · (3 · 4) = (2 · 3) · 4. | |
asymptote |
|
| A line that a graph of a curve approaches as closely as you wish. An asymptote is often represented by a dashed line on a graph. For example, the graph below has an asymptote at y = −3.
|
|
average |
|
| See “mean.” | |
axes |
|
| In a coordinate plane, two number lines that meet at right angles at the origin (0, 0). The x-axis runs horizontally and the y-axis runs vertically. See the example below.
|
|