A B C D E F G H IJK L M N O P Q R S T U V WXYZ
Making Connections Glossary
radius (plural: radii)  
  Of a circle: The line segment drawn from the center of a circle to a point on the circle.  Of a regular polygon: A line segment that connects the center of a regular polygon with a vertex.  The length of a radius is usually denoted  r.
random sample  
  A sample in which each item in the population or sample space has an equal chance of being selected.
range  
  The range of a set of data is the difference between the highest and lowest values.
rate  
  A ratio comparing two quantities, often a comparison of time.  For example, miles per hour.
ratio  
  A ratio compares two quantities by division.  A ratio may be written using a colon, but is more often written as a fraction.  For example, the comparison may be made of the ratio of female students in a particular school to the total number of students in the school.  This ratio could be written as 1521 : 2906 or as the fraction shown below.

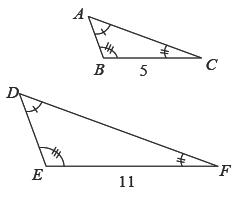
ratio of similarity  
  The ratio of any pair of corresponding sides of two similar figures.  This means that once it may be determined that two figures are similar, all of the pairs of corresponding sides of the figures have the same ratio.  For example, for the similar triangles  and  below, the ratio of similarity is .  The ratio of similarity may also be called the linear scale factor.
   
rational number  
  Numbers that may be expressed in the form , where a and b are integers and b ≠ 0.  For example, 0.75 is a rational number because 0.75 may be expressed in the form .
ray  
  A ray is part of a line that starts at one point and extends without end in one direction.  In the example below, ray 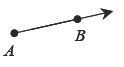 is part of line  that starts at A and contains all of the points of  that are on the same side of A as point B, including A.  Point A is the endpoint of .
   
real numbers  
  Irrational numbers together with rational numbers form the set of the real numbers.  For example, the following are all real numbers: .  All real numbers are represented on the number line.
reciprocals  
  The reciprocal of a nonzero number is its multiplicative inverse, that is, the reciprocal of x is .  For a number in the form , where  a  and  b  are non-zero, the reciprocal is .  The product of a number and its reciprocal is 1.  For example, the reciprocal of 12 is , because .
recompose  
  If a geometric figure is broken up into pieces and the pieces are put back together, possibly in a new way, then the figure is recomposed.  Similarly, if two or more numbers have been written as sums or differences in an expression and these numbers are put back together into sums or differences, then the expression is recomposed.  (Also see decompose.)
rectangle  
  A quadrilateral with four right angles.
reduce  
  To put a fraction into simplest form.
reflection  
  A transformation across a line that produces a mirror image of the original (pre-image) shape.  The reflection is called the “image” of the original figure.  The line is called a “line of reflection.”  Note that a reflection is also sometimes referred to as a “flip.”  See the example below.
   
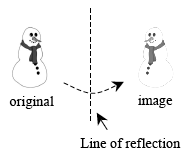regular polygon  
  A polygon is regular if the polygon is a convex polygon with congruent angles and congruent sides.  For example, the shape below is a regular hexagon.
   
repeating decimal  
  A repeating decimal is a decimal that repeats the same sequence of digits forever from some point onward.  For example, 4.56073073073… is a decimal for which the three digits 073 continue to repeat forever.  Repeating decimals are always the decimal expansions of rational numbers.
representative sample  
  A subset (group) of a given population with the same characteristics as the whole population.
rhombus  
 

A quadrilateral with four congruent sides.  (Also see equilateral.)
right angle  
  An angle that measures 90º.  A small square is used to note a right angle, as shown in the example below.
   
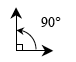right triangle  
  A triangle that has one right angle.  The side of a right triangle opposite the right angle is called the “hypotenuse,” and the two sides adjacent to the right angle are called “legs.” (See Pythagorean Theorem for a diagram.)
rigid transformations  
  Movements of figures that preserve the shape and size of the figures.
root fraction  
  The member of a family of fractions with the smallest integer values, the most simplified form.  For the fraction family  etc.,  is the root fraction.
rotation  
  A transformation that rotates (or turns) all of the points in the original (pre-image) figure the same number of degrees around a fixed center point (such as the origin on a graph).  The result is called the “image” of the original figure.  The point that the shape is rotated about is called the “center of rotation.”  To define a rotation, the measure of turn (in degrees) must be stated, the direction in which the shape is turned (such as clockwise or counter-clockwise), and the center of rotation.  See the example below.  Note that a rotation is also sometimes referred to as a “turn.”
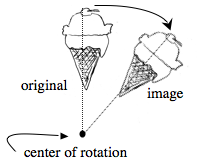